Blog

February 18, 2025
by GForce Software

Everything you need to know about tuning but were too afraid to ask. We’ll shed new light on oscillator tuning, detuning and fine-tuning in this article. Tuning oscillators is a basic part of synthesis. You likely set your oscillator pitches near the start of your patch-building process, and you probably don’t come back to change them later once these decisions have been baked in. You’ll likely know how unison detuning works, and know the great effects you can achieve with it, but we may be able to add to your knowledge of this effect. Plus, there’s another fundamental transformation lurking back at the oscillator stage that you might not have considered.

In this article, we’ll show you how tuning synth oscillators can affect the overall sound in ways you might not have realised. We’ll cover some basic principles like voice intervals and detuning, and then we’ll get into the more esoteric ways that pitch can have a fundamental effect on an overall synth sound later on in the article.

Just a heads-up—some of the the sound examples are raw to make it easier to discern the effect.

The Basics: Tuning Oscillators in Intervals

Even a basic synth will usually offer tuning for its oscillators – or one of them. In impOSCar3, Oscillator 1 always stays tuned to the MIDI note received, but we can tune Oscillator 2 against it by up to 1200 cents (an octave) higher or lower. Hold Shift to snap it to full 100-cent values (semitones).

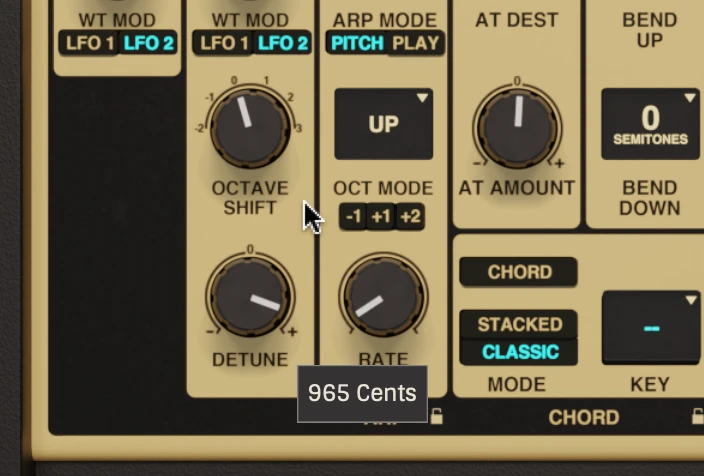
Doing this changes the character of the sound, and in the audio below, we go through key musical intervals, one at a time every four bars: 0 cents (unison), 400 cents (major third), 500 cents (fourth), 700 cents (fifth), 1200 cents (octave).

So which notes should you choose to tune your oscillators in? Octaves are the obvious choice, adding a second layer of the note, just higher, for a sweeter flavour. Getting a bit spicier, fifths (seven semitones) work very well to give a different tone but one that works with almost every note of the scale. Where fifths work, their equivalent inversions, fourths (five semitones), work just as well.

Major thirds (four semitones) and minor thirds (three semitones) work only in particular musical contexts, but can provide a very phat feel when they work. Adding an octave to any interval, such as choosing an octave-fifth (19 semitones) or octave-third (16 semitones) can sometimes fit in more easily with the wider piece of music.

Unison Detuning for Synth Oscillators

The next commonly known technique using tuning is in detuning synth oscillators – most commonly called Unison Detune.

The DIY way to achieve unison detune is to take oscillator A and fine-tune it slightly up or down (by about 7 cents to start, for example). Next, take oscillator B and fine-tune it the opposite way by the same amount.

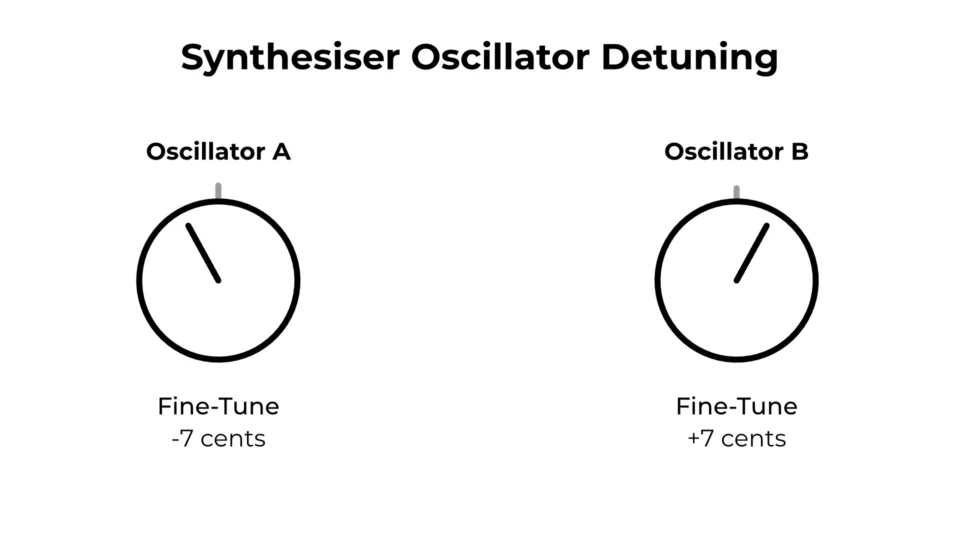
Below, -7 cents are applied to Oberheim OB-1 VCO1 using the Finetune knob, while +7 cents are applied to VCO2 using its own Finetune knob.

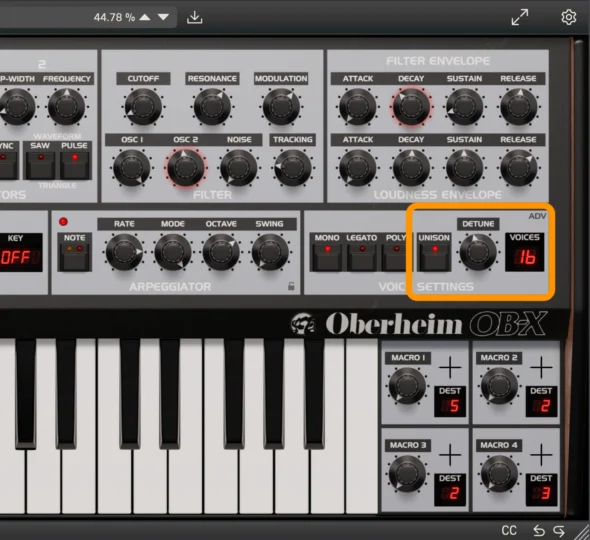
But more often, you’ll find a Unison control that does exactly this for you, like the one in OB-X, below, that operates across 16 voices and sounds huge! We turn it up throughout the audio example below.

What’s Actually Happening when Oscillators are Unison Detuned?

Oscillator detuning takes advantage of how we perceive sound. Our ears can only discriminate between two tones if their pitches are far apart enough. If the pitches are close enough together, the two tones will fuse into one, perceptually sounding like a note in the middle of both. If the pitches are too far, we’ll hear both notes separately. For more reading, search Critical Bands.

This principle still applies to complex sounds with lots of harmonics, as opposed to just working with pure sine waves.

This fact has been recognised for a long time, in fact. Most notes on a piano are actually made from two or three strings, tuned up and down but averaging at the note in the middle. This has been done in order to get a sound that’s as loud as the heavier, lower strings while still matching the impedance at the bridge where the strings transfer energy to the soundboard.

The Sweet Spot for Synth Oscillator Detune

So based on the idea of critical bands and the perceptual fusion of two tones, we can say that oscillator detuning has something of a sweet spot, where the two tones are perceived as one but thicker, rather than perceived as two separate tones.

In this example, the Detune parameter is increased from the start until the end of the audio file.

You can hear the synth start off with no detuning, then gradually get thicker while staying the same ‘note’. Then, the two oscillators really separate, each becoming nearly audible as separate tones by the end.

Here’s the thing: try listening to the same thing happening two octaves above…

In this case, it feels like these two higher tones become easier to hear as two tones, even earlier than the lower tones do. However, if this is determined by the workings of your inner ear and your particular neural wiring, then your mileage may vary!

The conclusion here? Synth detuning has its sweet spots in every situation. Just because a patch has a certain detune level, the effects change by playing lower or higher notes, so don’t be scared to tune it back.

Stabilising Tuned Oscillators with Just Ratios

There’s another thing that we can do to amend how a tuned oscillator pairing sounds.

You may have heard of Just Intonation, which is a system of tuning where instead of all 12 notes of the keyboard being equal, they’re related by ‘perfect’ ratios between their frequencies. In almost every synth, oscillators are tuned in semitones, not ratios, although FM synths do buck this trend.

You can get a better understanding of Just Intonation here, but for now, know that a ‘Just Fifth’ interval follows three frequency ratio of 3:2, so starting from the note 440Hz, its just fifth will be 660Hz (440 divided by two, multiplied by three). On the other hand, the fifth as we generally know it is 659.26Hz. That’s a small amount ‘off’, but it makes a difference. The only ‘just’ interval in a standard equal scale is the octave, with its 2:1 frequency. ratio

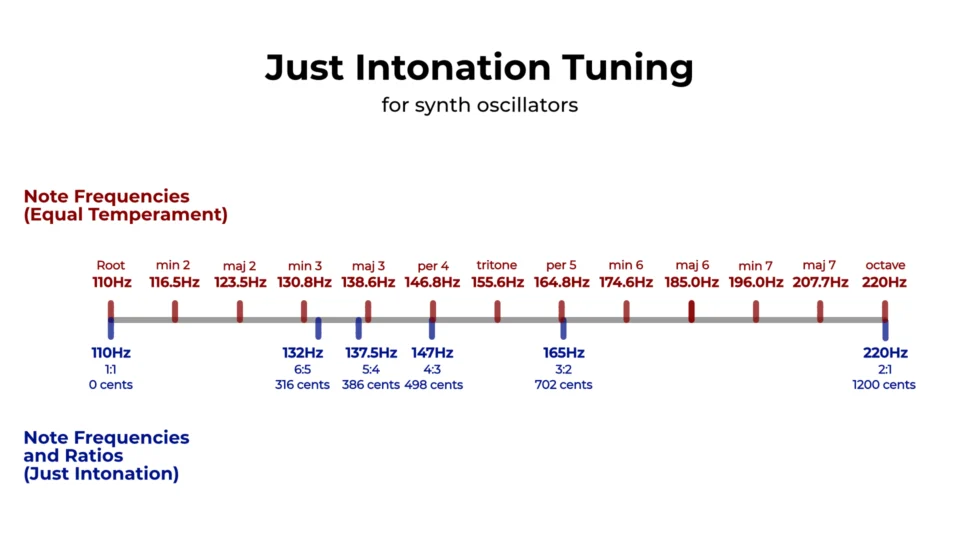
A just interval sounds smoother than its equivalent equally tempered interval. This happens because the two frequencies line up perfectly into a new repeating waveform, whereas a slight difference will end up causing amplitude modulation (beats) between the two notes.

You can hear the difference between a just fifth (+702 cents) and the equally temperature fifth (+700 cents) we usually experience. The difference may be subtle, but without the amplitude modulation, the oscillator tones are more stable – not that stability must always be your mission!

The effect is even more apparent when oscillators are stacked in thirds. A major third in equal temperament is 14 cents sharper than its equivalent ‘just’ interval with its ratio 5:4.

Tuning Synth Oscillators to Just Ratios

To help tune oscillators to just intervals, use the table above to see the number of cents difference each has with equal temperament. Most synth oscillator tuning will be performed in cents, so knowing the cent values of just ratios will help you to reach them in most synths. Our OB-1 synth is a great case for fine-tuning two oscillators.

Admittedly, the difference is largest for thirds over fifths and fourths, which are more likely to be used as layered oscillator pitches. Fifths and fourths work in tune with every scale note except one, whereas thirds vary between major and minor for any scale.

Fine-Tuning Differences Between Lower and Higher Notes
Intervals that aren’t just ratios create beats. What’s consistent about ‘mistuned’ intervals is their tuning in cents, relative to whatever frequency is being played. This means that as you go up the octaves, the frequency of beats gets faster. Our original example of a 440Hz led to beats at 0.75Hz, but down at 110Hz, these beats only waver every 0.2 seconds – that’s one cycle of amplitude modulation every five seconds.